Definition & Examples of static Equilibrium in Physics
statics, in physics, is the subdivision of mechanics that is concerned with the forces that act on bodies at rest under equilibrium conditions.
Basic theoretical information
Basics of statics
Statics is the section of mechanics that studies the equilibrium conditions of bodies. Equilibrium is a state of a body or system of bodies in which it does not move in a given frame of reference. There are three types of equilibrium:
- Stable balance. If the system is removed from the state of stable equilibrium, then it will spontaneously return to it, that is, when removing from the equilibrium position, a force arises, which returns the system to equilibrium. For this, it is necessary that the potential energy of the system in a state of stable equilibrium has a minimum value. Any physical system tends to a state of stable equilibrium. This means that any spontaneous process always runs with a decrease in potential energy.
- Unstable equilibrium. In this case, when removing from equilibrium, forces arise that lead the system out of equilibrium, and the system cannot spontaneously return to it. In a state of unstable equilibrium, the potential energy of the system has a maximum value.
- Indifferent balance. When removing from equilibrium in the system does not occur or returns, or leading in the direction of forces.
From the second law of Newton it follows that if the geometric sum of all external forces applied to a non-rotating body is zero, then the body is at rest or makes a uniform rectilinear motion (indeed, the acceleration of the body is zero). In this case, it is customary to say that the forces applied to the body counterbalance each other. When calculating the resultant force, all forces acting on the body can be applied to the center of mass. Center of mass (or center of gravity) – the point to which the force of gravity is applied, acting on the body.
In order for a non-rotating body to be in balance, it is necessary that the resultant of all forces applied to the body be equal to zero. In other words, the vector sum of all forces applied to the body must be equal to zero:
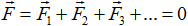
Moment of power. Rule of moments
If a body can rotate relative to a certain axis, then for its equilibrium, it is not enough that the resultant zero force of all forces is zero. The rotational action of a force depends not only on its magnitude, but also on the distance between the line of action of the force and the axis of rotation. The length of the perpendicular drawn from the axis of rotation to the line of action of force is called the shoulder of force .
To describe the causes of the rotation and the equilibrium conditions of the body in statics, a new concept is introduced – the moment of force. Product of the force module F on the shoulder d is called the moment of force M . Thus, the moment of force in statics is calculated by the formula:

Usually in physics the following rule of signs is used: if the force turns the body clockwise, then its moment is considered positive, and if against it, then the moment is negative. The moment of force can be zero if the force passes (by itself or by continuation) through the axis. Note: if you mix up and take the moment signs in reverse (clockwise with a minus sign, and counter-clockwise with a plus sign), then nothing terrible will happen. Therefore, it is important to remember that the moments of forces that rotate the body in different directions relative to the hour hand are taken with different signs.
Please note that the moment of force depends not only on the magnitude of the force, but also on its shoulder. Therefore, the same moment value can be obtained in two ways: take a large force and a small shoulder, or take a small force and a large shoulder. Conclusion: the more leverage, the less force must be applied to obtain the same result.
Rule of moments: a body having a fixed axis of rotation is in equilibrium if the algebraic sum of the moments of all forces applied to the body about this axis is zero:
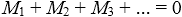
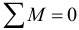
When recording this condition in the course of solving a particular problem of static, moments of forces must be recorded taking into account their signs. In the International System of Units (SI), the torques are measured in newton meters (N ∙ m).
Note: in the general case, when the body can move progressively and rotate, for balance it is necessary to fulfill both conditions: the equality to zero of the resultant force and the equality to zero of the sum of all moments.
Algorithm for solving problems on the rule of moments (static problems):
- Draw a picture. It should be remembered that the force of gravity acting on the body is depicted once. If the problem concerns a broken stick, it is more convenient to draw separately the forces of gravity acting on each part of the stick, considering the masses of the parts to be proportional to their lengths. Unlike dynamics, where forces are depicted from one point, in statics it is important to accurately indicate the point of application of force.
- Choose the axis of rotation at the point of application of the most unnecessary in the task of force or forces (the force that is not necessary to define and do not want because of the natural feeling of laziness). In this case, the shoulder (and, therefore, the moment) of this force will vanish regardless of its magnitude, and in further calculations this force can be completely ignored.
- Write the rule of moments on the given axis, forgetting about the rule of signs.
- If necessary, also write the condition according to which the resultant force is equal to zero.
- Express the desired power.
Levers and blocks
As you know from practice, sometimes it is necessary to change the direction of force, increase or decrease its value. This purpose is served by simple mechanisms: devices that convert the magnitude or direction of a force through mechanical phenomena. For all simple mechanisms, the golden rule of mechanics holds true : won in power – lost in movement (or vice versa) . This means that with increasing force due to some mechanism, displacement will inevitably be reduced. Consider the main types of simple mechanisms studied in school physics:
- Equal-arm lever (scales). A lever whose axis of rotation passes through its geometric center.
- Unequal shoulder lever. The lever is the axis of rotation which passes through an arbitrary point.
- Fixed unit. This is a disk with a fixed axis through which the thread has been transferred. Fixed block is used to change the direction of application of force. If there is no friction in the block, the thread is weightless, then the force of its tension before and after the block does not change. Thus, a fixed block does not give a gain in power, nor a loss in movement.
- Moving unit. This is a disk whose axis can move progressively. The movable block allows you to reduce the force by half, at the same time doubling the movement.
- Inclined plane. This device is used for weight lifting. At sufficiently small values of the angle of inclination and a small coefficient of friction, the force that must be applied to lift a certain body along an inclined plane can be significantly less than the body weight. Thus, lifting becomes easier. Naturally, in this case, in full accordance with the “golden rule”, the movement of the body increases.
Body center of gravity
Center of mass (or center of gravity) – the point to which the force of gravity is applied, acting on the body. In general, the center of gravity may not lie inside the body, but go beyond it (for example, various curved long objects, rings, half rings, and so on).
Consider the basic methods for determining the position of the center of mass of bodies for some specific cases that arise when solving problems in statics:
1. In homogeneous bodies of regular shape (balls, rectangles, rods), the center of gravity coincides with the geometric center. It should be remembered that the center of gravity of a homogeneous triangular plate lies at the intersection point of its medians. For homogeneous symmetric bodies, the center of gravity is always located on the axis of symmetry.
2. Determining the position of the center of gravity of a two-body system with known centers of gravity. Here you can use the remarkable property of the center of gravity. By supporting the center of gravity, we will ensure the balance of the body. Thus, the center of gravity of a two-body system lies on the segment connecting their centers of gravity, and divides it in the ratio inverse to the ratio of the masses of the bodies:

where: l 1 is the distance from the center of mass to the body with the mass m 1 , and l 2 – to the body with the mass m 2 .
3. Determining the position of the center of gravity of any system of bodies with known positions of the centers of gravity. It is necessary to enter the coordinate system (of course, choose the origin of coordinates at a point relative to which it is necessary to calculate the position of the center of gravity), determine in it the coordinates of the centers of gravity of all bodies and find the coordinates of the center of gravity of the system using the formula:
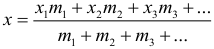
Similar equations are obtained for the remaining coordinate axes, if they need to be considered in the problem (the variable x is simply changed to y or z, respectively).
4. A uniform body of the correct form with cut of the correct form. The easiest thing to do is to reduce the task to the reverse: mentally insert the neckline back and get the body of the correct shape with a known position of the center of gravity. Next, to present it in the form of two bodies: the terrible with the notch and the very notch. And now everything is simple. At one of the bodies (notch) we know the position of the center of gravity. The other – no. But we know the position of the center of gravity of the two-body system. Making an equation to determine the common center of gravity, we obtain an expression with one unknown – the center of gravity of the body with a notch. Solving the equation we get the desired answer.
5. The Pappus Theorem. It is used to determine the position of the center of gravity of a flat plate, which, when rotated around a certain axis, forms a body with easily calculated volume. It is necessary to mentally rotate the plate by one turn, draw a picture and apply the theorem:
The formulation of the theorem: the volume of the body obtained by rotating the plate is equal to the product of its area and the path traveled by the center of gravity during rotation