Molecular physics formulas, impact Factor
Basic Theoretical Information
The main provisions of the ICB
Molecular-kinetic theory refers to the theory of the structure and properties of a substance based on the idea that atoms and molecules exist as the smallest particles of a chemical substance. The basis of molecular-kinetic theory is based on three main points:
- All substances – liquid, solid and gaseous – are formed from the smallest particles – molecules, which themselves consist of atoms (“elemental molecules”). Chemical molecules can be simple and complex and consist of one or more atoms. Molecules and atoms are electrically neutral particles. Under certain conditions, molecules and atoms can acquire an additional electrical charge and turn into positive or negative ions (respectively, anions and cations).
- Atoms and molecules are in continuous chaotic motion and interaction, the speed of which depends on temperature, and the character – of the state of aggregation of matter.
- Particles interact with each other by forces that have an electrical nature. The gravitational interaction between particles is negligible.
An atom is the smallest chemically indivisible particle of an element (an atom of iron, helium, or oxygen). A molecule is the smallest particle of a substance that preserves its chemical properties. A molecule consists of one or more atoms (water – H 2 O – 1 oxygen atom and 2 hydrogen atoms). An ion is an atom or molecule that has one or more electrons extra (or not enough electrons).
Molecules are extremely small. Simple monatomic molecules have a size of the order of 10–10 m. Complex polyatomic molecules can be hundreds or thousands of times larger.
The random chaotic movement of molecules is called thermal motion. The kinetic energy of thermal motion increases with increasing temperature. At low temperatures, molecules condense into liquid or solid. With increasing temperature, the average kinetic energy of the molecule becomes larger, the molecules scatter, and the gaseous matter is formed.
In solids, molecules make random fluctuations around fixed centers (equilibrium positions). These centers can be located in space in an irregular manner (amorphous bodies) or form ordered bulk structures (crystalline bodies).
In liquids, molecules have significantly more freedom for thermal movement. They are not tied to specific centers and can move throughout the entire volume of fluid. This explains the fluidity of liquids.
In gases, the distances between molecules are usually much larger than their size. The interaction forces between molecules at such large distances are small, and each molecule moves along a straight line until another collision with another molecule or with the vessel wall. The average distance between air molecules under normal conditions is of the order of 10–8 m, that is, hundreds of times larger than the size of the molecules. The weak interaction between molecules explains the ability of gases to expand and fill the entire volume of the vessel. In the limit, when the interaction tends to zero, we come to the idea of an ideal gas.
An ideal gas is a gas whose molecules do not interact with each other, with the exception of elastic collision processes, and are considered to be material points.
In molecular kinetic theory, the amount of a substance is considered to be proportional to the number of particles. The unit of quantity of a substance is called a mole (mol). A mole is a quantity of a substance containing as many particles (molecules) as there are atoms in 0.012 kg of carbon 12 C. A carbon molecule consists of one atom. Thus, one and the same mole of any substance contains the same number of particles (molecules). This number is called Avogadro’s constant: N A = 6.022 · 10 23 mol –1.
Avogadro’s constant is one of the most important constants in the molecular-kinetic theory. The amount of a substance is defined as the ratio of the number N of particles (molecules) of a substance to Avogadro’s constant N A, or as the ratio of mass to molar mass:
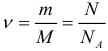
The weight of one mole of a substance is called a molar mass M. The molar mass is equal to the product of the mass m 0 of one molecule of a given substance by the Avogadro constant (that is, by the number of particles in one mole). Molar mass is expressed in kilograms per mole (kg/mol). For substances whose molecules consist of one atom, the term atomic mass is often used. In the periodic table, the molar mass is in grams per mole. Thus, we have another formula:

where: M is the molar mass, N A is the Avogadro number, m 0 is the mass of one particle of a substance, and N is the number of particles of a substance contained in the mass of a substance m. In addition, we need the concept of concentration(the number of particles per unit volume):

Recall also that the density, volume, and body weight are related by the following formula:

If the problem is a mixture of substances, then we speak about the average molar mass and the average density of the substance. As in the calculation of the average speed of uneven motion, these values are determined by the total mass of the mixture:


Do not forget that the total amount of a substance is always equal to the sum of the quantities of substances in the mixture, and you must be careful with the volume. The volume of the mixture of gases is not equal to the sum of the volumes of gases entering the mixture. So, 1 cubic meter of air contains 1 cubic meter of oxygen, 1 cubic meter of nitrogen, 1 cubic meter of carbon dioxide, etc. For solids and liquids (unless otherwise specified in the condition), we can assume that the volume of the mixture is equal to the sum of the volumes of its parts.
Basic equation MKT ideal gas
As it moves, gas molecules continuously collide with each other. Because of this, the characteristics of their movements change, therefore, speaking of impulses, velocities, and kinetic energies of molecules, we always mean the mean values of these quantities.
The number of collisions of gas molecules in normal conditions with other molecules is measured millions of times per second. If we neglect the size and interaction of molecules (as in the ideal gas model), then we can assume that between successive collisions molecules move uniformly and straightforwardly.
Naturally, flying up to the wall of the vessel in which the gas is located, the molecule experiences a collision with the wall. All collisions of molecules with each other and with vessel walls are considered absolutely elastic collisions of balls. When a molecule collides with a wall, the impulse of the molecule changes, so a force acts on the molecule from the side of the wall (remember Newton’s second law).
But according to the third law of Newton with exactly the same force directed in the opposite direction, the molecule acts on the wall, exerting pressure on it. The combination of all the impacts of all molecules on the vessel wall leads to gas pressure.
The gas pressure is the result of collisions of molecules with the walls of the vessel. If there is no wall or any other obstacle for molecules, then the very concept of pressure loses its meaning. For example, it is completely unscientific to talk about pressure in the center of the room, because there the molecules do not press on the wall.
Why, then, having placed a barometer there, we are surprised to find that it shows some pressure? Right! Because the barometer itself is the very wall on which the molecules are pressed. It is completely unscientific to talk about pressure in the center of the room because there the molecules do not press on the wall. Why, then, having placed a barometer there, we are surprised to find that it shows some pressure? Right! Because the barometer itself is the very wall on which the molecules are pressed.
It is completely unscientific to talk about pressure in the center of the room because there the molecules do not press on the wall. Why, then, having placed a barometer there, we are surprised to find that it shows some pressure? Right! Because the barometer itself is the very wall on which the molecules are pressed.
Since pressure is a consequence of the impact of molecules on the vessel wall, it is obvious that its value should depend on the characteristics of individual molecules (of average characteristics, of course, you remember that the speeds of all molecules are different). This dependence is expressed by the basic equation of the molecular kinetic theory of an ideal gas :
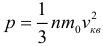
where: p is the gas pressure, n is the concentration of its molecules, m 0 is the mass of one molecule, v q is the mean square velocity (note that in the equation itself stands the square of the mean square velocity). The physical meaning of this equation is that it establishes a connection between the characteristics of the entire gas (pressure) and the motion parameters of individual molecules, that is, the relationship between the macro and microworld.
Consequences of the basic equation
As already noted in the previous paragraph, the rate of thermal movement of molecules is determined by the temperature of the substance. For an ideal gas, this dependence is expressed by simple formulas for the mean square velocity of movement of gas molecules:
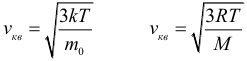
where: k = 1.38 ∙ 10 –23 J / K is the Boltzmann constant, and T is the absolute temperature. Immediately make a reservation that further in all tasks you must, without hesitation, convert temperature to kelvins from degrees Celsius (except for problems on the heat balance equation). The law of three permanent :
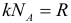
where: R = 8.31 J / (mol K) is the universal gas constant. The following important formula is the formula for the average kinetic energy of the translational motion of gas molecules :
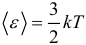
It turns out that the average kinetic energy of the translational motion of molecules depends only on temperature, and is the same at a given temperature for all molecules. Finally, the most important and frequently used consequences of the basic ICB equation are the following formulas:

Temperature measurement
The concept of temperature is closely related to the concept of thermal equilibrium. Bodies in contact with each other can exchange energy. The energy transferred from one body to another through thermal contact is called the amount of heat.
Thermal equilibrium is a state of a system of bodies in thermal contact, in which heat transfer from one body to another does not occur, and all macroscopic parameters of the bodies remain unchanged. Temperature is a physical parameter that is the same for all bodies in thermal equilibrium.
To measure the temperature, physical devices are used – thermometers, in which the value of temperature is judged by the change in a physical parameter. To create a thermometer, it is necessary to select a thermometric substance (for example, mercury, alcohol) and a thermometric quantity characterizing the property of a substance (for example, the length of a mercury or alcohol column).
In various designs of thermometers, various physical properties of a substance are used (for example, a change in the linear dimensions of solids or a change in the electrical resistance of conductors when heated).
Thermometers must be calibrated. For this, they are brought into thermal contact with bodies whose temperatures are assumed to be given. Most often, simple natural systems are used in which the temperature remains unchanged, despite the heat exchange with the environment – a mixture of ice and water and a mixture of water and steam at boiling at normal atmospheric pressure.
On the temperature scale of Celsius, the melting point of ice is assigned a temperature of 0 ° C, and the boiling point of water: is 100 ° C. The change in the length of the liquid column in the capillaries of the thermometer for one-hundredth of the length between 0 ° C and 100 ° C is taken equal to 1 ° C.
The English physicist U. Kelvin (Thomson) 1848 proposed to use the point of zero gas pressure to build a new temperature scale (Kelvin scale). In this scale, the unit of measurement of temperature is the same as in the Celsius scale, but the zero point is shifted:

At the same time, a temperature change of 1 ° C corresponds to a temperature change of 1 K. The temperature changes on the Celsius and Kelvin scale are equal. In the SI system, it is accepted to call the Kelvin temperature measurement unit Kelvin and denote it by K. For example, the room temperature T C = 20 ° C on the Kelvin scale is T К = 293 K. The Kelvin temperature scale is called the absolute temperature scale. It turns out to be the most convenient when constructing physical theories.
The equation of state of an ideal gas or the Clapeyron-Mendeleev equation
The equation of state of an ideal gas is another consequence of the basic ICT equation and is written in the form:
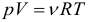
This equation establishes the relationship between the basic parameters of the state of an ideal gas: pressure, volume, amount of matter, and temperature. It is very important that these parameters are interrelated, a change in any of them will inevitably lead to a change in at least one more. That is why this equation is called the ideal gas equation of state. It was opened first to one mole of gas by Clapeyron, and subsequently generalized to the case of a larger number of moles by Mendeleev.
If the gas temperature is T n = 273 K (0 ° C), and the pressure p n = 1 atm = 1 · 10 5 Pa, then it is said that the gas is under normal conditions.
Gas laws
Solving problems for calculating gas parameters is greatly simplified if you know which law and which formula to apply. So, consider the basic gas laws.
1. The law of Avogadro. One mole of any substance contains the same number of structural elements equal to the Avogadro number.
2. Dalton’s law. The pressure of the mixture of gases is equal to the sum of the partial pressures of the gases entering this mixture:
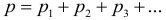
The partial pressure of a gas is the pressure that it would produce if all other gas suddenly disappeared from the mixture. For example, air pressure is equal to the sum of the partial pressures of nitrogen, oxygen, carbon dioxide, and other impurities. In addition, each of the gases in the mixture occupies the entire volume provided to it, that is, the volume of each of the gases is equal to the volume of the mixture.
3. The law of Boyle-Mariotta. If the mass and temperature of the gas remain constant, then the product of the gas pressure and its volume does not change, therefore:

The process that takes place at a constant temperature is called isothermal. Please note that such a simple form of the Boyle-Mariotte law is carried out only under the condition that the mass of the gas remains unchanged.
4. The Law of Gay-Lussac. The Gay-Lussac Act itself is not particularly valuable when preparing for exams, therefore, we give only a consequence of it. If the mass and pressure of the gas remain constant, then the ratio of the volume of gas to its absolute temperature does not change, therefore:

The process that takes place at constant pressure is called isobaric or isobaric. Please note that this simple form of the Gay-Lussac law is carried out only on condition that the mass of the gas remains unchanged. Do not forget about the transfer of temperature from degrees Celsius to kelvin.
5. Law of Charles. Like the law of Gay-Lussac, the law of Charles in the exact formulation is not important for us, therefore we will give only a consequence of it. If the mass and volume of gas remain constant, the ratio of gas pressure to its absolute temperature does not change, therefore:
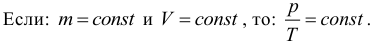
The process that occurs at a constant volume is called isochoric or isochoric. Please note that such a simple form of Charles law is carried out only under the condition that the mass of the gas remains unchanged. Do not forget about the transfer of temperature from degrees Celsius to kelvin.
6. Universal gas law (Clapeyron). With a constant mass of gas, the ratio of the product of its pressure and volume to temperature does not change, therefore:

Please note that the mass should remain unchanged, and do not forget about Kelvin.
So, there are several gas laws. We list the signs that you need to apply one of them when solving a problem:
- Avogadro’s law is applied in all tasks when it comes to the number of molecules.
- Dalton’s law applies to all problems in which it is a question of a mixture of gases.
- Charles’s law is used in problems when the volume of gas remains unchanged. This is usually either explicitly stated, or the words “gas in a closed vessel without a piston” are present in the problem.
- The Gay-Lussac Act is applied if the gas pressure remains unchanged. Search for the words “gas in a vessel closed by a moving piston” or “gas in an open vessel.” Sometimes nothing is said about the vessel, but by the condition, it is clear that it communicates with the atmosphere. Then it is considered that the atmospheric pressure always remains the same (unless otherwise stated in the condition).
- Boyle-Mariotte law. It’s hardest here. Well, if the problem says that the temperature of the gas is unchanged. Slightly worse if the condition contains the word “slow.” For example, the gas is slowly compressed or slowly expanded. Even worse, it is said that the gas is closed by a heat-conducting piston. Finally, it’s very bad if nothing is said about the temperature, but from the condition, we can assume that it does not change. Usually, in this case, the students apply the Boyle-Mariotte law of hopelessness.
- Universal gas law. It is used if the mass of the gas is constant (for example, the gas is in a closed vessel), but by the condition, it is clear that all other parameters (pressure, volume, temperature) change. In general, often instead of the universal law, you can apply the Clapeyron-Mendeleev equation, and you will get the correct answer, only in each formula, you will write two extra letters.
Isoprocess graphic image
In many sections of physics, the dependence of the quantities on each other is conveniently depicted graphically. This simplifies the understanding of the relationship between the parameters occurring in the system of processes. Such an approach is very often used in molecular physics. The main parameters describing the state of an ideal gas are pressure, volume, and temperature.
Graphical method of solving problems and consists in depicting the relationship of these parameters in different gas coordinates. There are three main types of gas coordinates: ( p; V ), ( p; T ), and ( V; T). Note that these are only basic (most common types of coordinates). The fantasy of the compilers of the tasks and tests is not limited, therefore you can meet any other coordinates. So, we will depict the main gas processes in the main gas coordinates.
Isobaric process (p = const)
The isobaric process is a process that proceeds at a constant pressure and mass of gas. As follows from the equation of the state of an ideal gas, in this case, the volume changes in direct proportion to temperature. Graphs of the isobaric process in coordinates p – V; V – T and p – T have the following form:
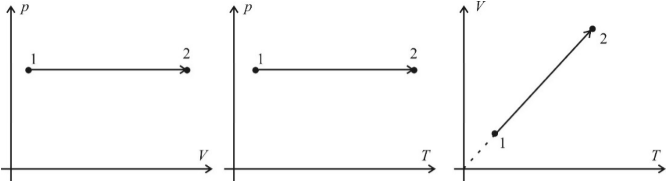
Note that the continuation of the graph in V – T coordinates is directed exactly to the origin, however, this graph can never start directly from the origin, since at very low temperatures the gas turns into liquid, and the dependence of volume on temperature changes.
Isochoric process (V = const)
An isochoric process is the process of heating or cooling gas at a constant volume and providing that the amount of the substance in the vessel remains unchanged. As follows from the equation of the state of an ideal gas, under these conditions, the gas pressure changes in direct proportion to its absolute temperature. Graphs of the isochoric process in the coordinates p – V ; p – T and V – T have the following form:
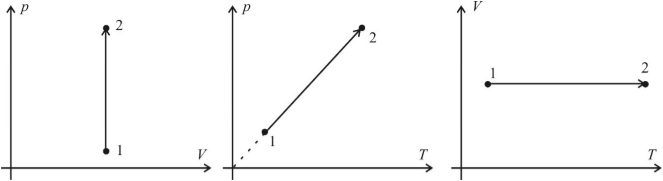
Note that the continuation of the graph in p – T coordinates are directed exactly to the origin, but this graph can never start directly from the origin since the gas turns into liquid at very low temperatures.
Isothermal process (T = const)
An isothermal process is a process that proceeds at a constant temperature. From the equation of the state of an ideal gas, it follows that at a constant temperature and a constant amount of a substance in a vessel, the product of the gas pressure and its volume must remain constant. Graphs of the isothermal process in the coordinates p – V ; p – T and V – T have the following form:
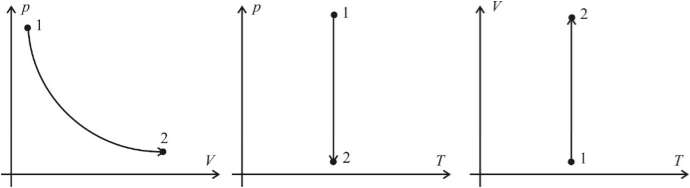
Note that when performing tasks on graphics molecular physics does not require special precision in postponing the coordinates of the respective axes (e.g., the coordinates of p 1 and p 2 of the two states of the gas in the system p ( V ) coincide with the coordinates of p 1 and p 2 of these states in the p ( T ) system. Firstly, these are different coordinate systems in which a different scale can be chosen, and secondly, this is an extra mathematical formality that distracts from the main thing – from analyzing the physical situation. was true.
Geoprocessing
In problems of this type, all three basic parameters of the gas change: pressure, volume, and temperature. Only gas mass remains constant. The simplest case is when the problem is solved head-on with the help of the universal gas law. It is a bit more difficult if you need to find the equation of a process describing a change in the state of a gas, or analyze the behavior of gas parameters according to this equation.
Then we must act in this way. Write down this process equation and the universal gas law (or the Klapeyron-Mendeleev equation, which is more convenient for you) and successively exclude unnecessary quantities from them.
The change in the amount or mass of the substance
In essence, there is nothing difficult in such tasks. It is only necessary to remember that the gas laws are not fulfilled, since in the formulations of any of them they are written: “at constant mass”. Therefore, we act simply. Write the Clapeyron-Mendeleev equation for the initial and final states of the gas and solve the problem.
Partitions or pistons
Gas problems are again applied in problems of this type, and the following remarks should be taken into account:
- First, the gas does not pass through the partition, that is, the mass of gas in each part of the vessel remains unchanged, and thus, gas laws are fulfilled for each part of the vessel.
- Secondly, if the partition is heat-conducting, then when the gas is heated or cooled in one part of the vessel, the gas temperature in the second part will remain unchanged.
- Thirdly, if the partition is movable, then the pressures on both sides are equal at any given time (but this pressure is equal on both sides may vary with time).
- And then we write the gas laws for each gas separately and solve the problem.
Gas laws and hydrostatics
The specificity of the tasks is that the pressure will have to take into account the “overhangs” associated with the pressure of the liquid column. What could be the options here:
- The vessel with gas is immersed in water. The pressure in the vessel will be p = p atm + ρgh, where: h is the depth of immersion.
- The horizontal tube is sealed from the atmosphere by a mercury column (or other liquid). The gas pressure in the tube is exactly equal to p = p atm atmospheric since the horizontal mercury column does not exert pressure on the gas.
- The vertical tube with gas is closed on top with a mercury column (or other liquid). Gas pressure in the tube: p = p atm + ρgh, where: h is the height of the mercury column.
- The vertical narrow tube with gas is turned with its open end down and locked with a column of mercury (or other liquid). Gas pressure in the tube: p = p atm – ρgh, where: h is the height of the mercury column. The “-” sign is placed because mercury does not compress but stretches the gas. Often, students ask why mercury does not flow out of the tube. Indeed, if the tube were wide, the mercury would be glass down the walls. And so, since the tube is very narrow, the surface tension on the mercury allows to burst in the middle and let the air in, and the gas pressure inside (less than atmospheric) keeps the mercury from flowing out.
Once you have managed to correctly record the gas pressure in the tube, apply any of the gas laws (as a rule, Boyle-Mariotte, since most of these processes are isothermal, or universal gas law). Apply the chosen law for gas (in no case for liquid) and solve the problem.
Thermal expansion of bodies
With increasing temperature, the intensity of the thermal motion of particles of matter increases. This leads to the fact that the molecules are more “actively” repelling each other. Because of this, most bodies increase their size when heated. Do not make a typical mistake, the atoms themselves and the molecules do not expand when heated. Only empty spaces between molecules increase. The thermal expansion of gases is described by the Gay-Lussac law. Thermal expansion of liquids is subject to the following law:

where: V 0 – the volume of liquid at 0 ° С, V – at temperature t, γ – coefficient of volume expansion of the liquid. Please note that all temperatures in this topic must be taken in degrees Celsius. The coefficient of volume expansion depends on the type of fluid (and on temperature, which is not taken into account in most tasks). Please note that the numerical value of the coefficient, expressed in 1 / ° C or 1 / K, is the same, since heating a body by 1 ° C is the same as heating it by 1 K (and not by 274 K).
Three formulas are used to expand solids, describing the change in linear dimensions, area, and volume of the body:
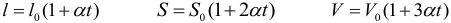
where: l 0, S 0, V 0 – respectively, the length, surface area, and volume of the body at 0 ° C, α – the coefficient of linear expansion of the body. The coefficient of linear expansion depends on the type of body (and temperature, which is not taken into account in most tasks) and is measured at 1 / ° C or 1 / K.
