Irrational Numbers – Definition, List, Properties, Examples of problems with solutions
Some recommendations for solving irrational equations and systems
There are two equivalent methods for solving irrational equations with square roots:
- The method of equivalent transitions (taking into account the TLD). At the same time, in order to correctly record the range of permissible values, in the general case it is necessary to require the nonnegativity of all the radicals, as well as expressions that are equal to square roots (if they can be expressed algebraically from the equation).
- The method of transition to the equation-effect (excluding DHS). In this method, root substitution checking is required.
Honestly speaking, in irrational equations it is sometimes so difficult to correctly write TLDs that even if you try to do this, the roots are still better checked by substitution, especially if the roots are integers.
Note the very common mistake – if you solve an equation like:
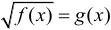
Then, when writing TLDs, it is necessary to require the non-negativity of the right part, that is, to impose a condition:

Moreover, it is necessary to understand that this condition should be additionally added to DHS, even if you came to a similar equation after several transformations (squaring), and not only in the case when the equation initially looked like.
In the irrational equations, the following remark becomes especially relevant: in order for the product of several factors to be equal to zero, it is necessary that at least one of them equals zero, and the others exist . When factors are roots, and not just parentheses as in rational equations, they often may not exist. So errors occur.
If there are many roots in an irrational equation, then it is highly desirable to move the roots from right to left or vice versa before squaring this equation so that each of the parties has exactly the sum of the roots, that is, a knowingly positive expression. If, for some reason, you decide to square the root difference (i.e., an expression whose sign is unknown), then be prepared to get a few extraneous roots. In this case, it is necessary to check all the roots by substitution, because it is most likely that it will not work out correctly to write TLDs.
If the irrational equation has a root in the root, then it will be necessary to squander this equation several times, while the main thing is to understand that, in accordance with the conditions outlined above, with each such erection, more and more new conditions can be obtained for DHS. In such equations, if possible, it is better to check the roots by substitution.
When solving irrational equations, it is often convenient to use a replacement. In this case, the main thing to remember is that after introducing a substitution into some equation, this equation should:
- first, become simpler;
- secondly, no longer contain the original variable.
In addition, it is important not to forget to perform a reverse replacement, i.e. after finding the values for the new variable (for replacement), write instead of replacement for what it is equal through the original variable, equate this expression with the values found for replacement and again solve the equations.
When solving systems of irrational equations with two unknowns, it is often enough to act according to the standard scheme. Namely, to express one of the variables from one of the equations and substitute this expression instead of the corresponding variable in another equation. Then a certain irrational equation with one unknown will be obtained, which should then be solved taking into account all the rules for solving irrational equations. The value of the first variable must then be found using its expression through the variable already found.
When solving systems of irrational equations with a large number of variables, it is also often sufficient to use the substitution method. Also, the method of changing variables often helps in solving systems of irrational equations. It should be understood that after the introduction of the replacement of variables into the system:
- first, it must again be simplified;
- secondly, new variables should be the same as old ones;
- thirdly, the system should no longer contain old variables;
- Fourthly, you need to remember to perform a reverse replacement.
Basic properties of degrees
When solving irrational equations, it is necessary to remember many properties of degrees and roots. We list below the main ones. Mathematical degrees have several important properties:
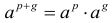

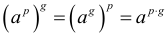
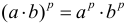
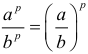

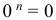
The last property holds only for n > 0. Zero can only be raised to a positive power. Well, the main property of the negative degree is written as follows:
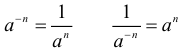
Basic properties of mathematical roots
The mathematical root can be represented in the form of an ordinary degree, and then use all the properties of the degrees given above. To represent the mathematical root in the form of a degree, use the following formula:

Nevertheless, it is possible to write out separately a number of properties of mathematical roots, which are based on the properties of the degrees described above:

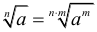
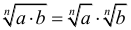

For arithmetic roots, the following property holds (which can also be considered the definition of a root):
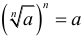
The latter is true: if n is odd, then for any a ; if n is even, then only if a is greater than or equal to zero. For the root of odd degree , the following equality is also fulfilled (from the root of the odd degree, one can remove the minus sign):

Since the value of a root of even degree can only be non-negative , for such roots there is the following important property:

So you should always remember that under the root of an even degree only a non-negative expression can stand, and the root itself is also a non-negative expression. In addition, it should be noted that if you use a record with a mathematical root icon, then the exponent of this root can only be an integer, and this number must be greater than or equal to two:

Basic properties of square root
The square root is the second degree mathematical root:
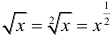
A square root can only be extracted from a non-negative number. The value of the square root is also always non-negative:
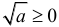
For a square root, there are two important properties that are important to remember very well and not to confuse:
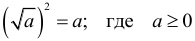

If there are several factors under the root, then the root can be extracted from each of them separately. It is important to understand that each of these factors separately (and not just their product) must be non-negative:
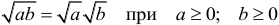
Note the other use case of the last property. If under the square root there is a product of two negative values (i.e., by the total, the value is positive, which means the root exists), then this root is factorized as follows:
