Modules Definition in Mathematics and Their Examples
In mathematics, a module is a generalization of the notion of vector space in which the field of scalars is replaced by a ring. The concept of module generalizes also the notion of the abelian group since the abelian groups are exactly the modules over the ring of integers.
Basic theoretical information
Basic information about the module
The definition of a module can be given as follows: The absolute value of a (module) is the distance from a point representing a given number a on a coordinate line, to the origin of coordinates. From the definition it follows that:

Thus, in order to open a module, it is necessary to determine the sign of a submodular expression. If it is positive, then you can simply remove the sign of the module. If the submodular expression is negative, then it needs to be multiplied by a minus, and the module sign, again, no longer writes.
The main properties of the module:

Some methods for solving equations with modules
There are several types of equations with a module for which there is a preferred solution. However, this method is not the only one. For example, for an equation of the form:
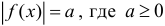
The preferred solution is to go to the aggregate:
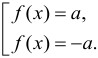
And for equations of the form:

You can also go to an almost similar set, but since the module takes only positive values, then the right side of the equation should be positive. This condition should be added as a general limitation to the whole example. Then we get the system:
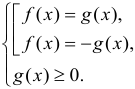
Both of these types of equations can be solved in another way: by appropriately revealing the module on the intervals where the submodule expression has a definite sign. In this case, we will receive a combination of two systems. We give a general view of the solutions obtained for both types of equations given above:

To solve equations containing more than one module, the interval method is applied , which consists of the following:
- First we find the points on the numerical axis in which each of the expressions under the module vanishes.
- Next, we divide the entire numerical axis into the intervals between the obtained points and examine the sign of each of the submodular expressions on each interval. Note that to determine the sign of an expression, it is necessary to substitute in it any value of x from the interval, except for the boundary points. Choose those x values that are easy to substitute.
- Further, on each obtained interval, we open all the modules in the initial equation in accordance with their signs on the given interval and solve the obtained ordinary equation. In the final answer we write out only those roots of this equation that fall into the studied interval. Once again: we carry out such procedure for each of the received intervals.