Exponential equations, systems and inequalities
Exponential equations are equations in which variables occur as exponents. For example, exponential equations are in the form ax=by. To solve exponential equations with the same base, use the property of equality of exponential functions. If b is a positive number other than 1, then bx=by if and only if x=y.
Basic theoretical information
Range of allowable values in exponential functions
When solving problems of this topic, it is necessary to remember very well all the properties of the degrees and mathematical roots (in particular, the square root ), studied earlier. Let us dwell additionally on some properties of power and exponential functions that relate to their domain of admissible values (DHS). Consider a function of the form:

Such a function, strictly speaking, is neither exponential nor exponential. Nevertheless, in her example, it is possible to demonstrate well the various possible options for DHS. And there are three such options:
- If f ( x )> 0, then in this case g ( x ) can take any values;
- It is possible that f ( x ) = 0 provided that: g ( x )> 0 – pay attention and remember: zero cannot be raised to a negative degree (this is equivalent to division by zero), and zero to zero does not exist. Thus, a zero can only be in a positive degree , while a zero in any positive degree gives zero;
- Finally, f ( x ) can take negative values, provided that g ( x ) takes integer values. Thus, negative numbers can be raised only to “integer” degrees .
Let us dwell on the first of these properties. It says that positive numbers and functions can be raised to any power . There is also a reverse requirement: a number or function that is raised to a rational degree must be non-negative . Thus, the entry:

Meaning expression:
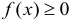
But, again, in the case of equality of the function to zero, it is always necessary to make sure separately that the degree was positive, since zero can only be raised to a positive power. Therefore, in the following, we will consider exponential equations and inequalities only with positive bases of degrees.
However, we note an important feature of the application of this property. The fact is that if a record with the designation of a mathematical root is used, then the root expression should not always be non-negative. We know that a negative expression can also stand under the root of an odd degree. Thus, in the record type:

A radical expression can take any value. But in the seemingly equivalent record of the following form:

The root expression again must be non-negative:
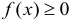
We also note another important property appearing when applying the notation of a mathematical root. So, if you use an entry with the mathematical root icon, then the exponent of this root can only be an integer, and this number must be greater than or equal to two:

Recommendations for solving exponential equations and systems
Consider the exponential equations in which the bases of all degrees are positive numbers that are not equal to one. In this case, the exponents can take all possible values, and at the same time such equations make sense. In the simplest cases, such exponential equations can be reduced by algebraic transformations to two types of equations. The first of these is the case when the left and right sides of the equation can be reduced to the same base. In this case, the converted equation will look like this:
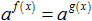
And his solution is sought by the transition to the following rational equation:

The second standard type of exponential equations is when both sides of the equation can be reduced to the same exponent, but for different reasons:
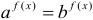
The only possible solution to this equation is:
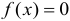
When solving exponential equations that cannot be reduced to one of the above equations, the method of changing variables is also actively used . As usual, when applying this method, one should remember that after introducing a replacement, the equation should simplify and no longer contain the old unknown. Also, do not forget to perform the reverse substitution of variables.
Separately, we will focus on the algorithm for solving very common homogeneous exponential equations . In general, homogeneous equations have the form:

Here A, B and C are numbers that are not equal to zero, and f ( x ) and g ( x ) are some exponential functions. Homogeneous equations are solved as follows: divide the entire equation by g 2 ( x ) and get:

Replace variables:

And solve the quadratic equation:
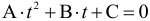
Having obtained the roots of this equation, we do not forget to perform the inverse replacement, and also to check the final roots for compliance with the LDL, if such was the case.
Sometimes when solving exponential equations one also has to use the graphical method . This method consists in constructing graphs of functions on the same coordinate plane as functions on the left and right sides of the equation, and then finding the coordinates of their intersection points in the drawing. Thus obtained roots must be verified by substitution into the original equation.
When solving systems of exponential equations, it is often necessary to try first in each of the equations of the system to move from the exponential equation to the usual rational one. To do this, each of the exponential equations is reduced to the same exponent or to the same base and go to rational equations as shown above. Then you need to solve a system of rational equations with one of the previously studied methods (usually by substitution). If this algorithm does not work, then you need to try to apply the method of division, change of variables or some other method directly to the system of exponential equations.
Recommendations for solving indicative inequalities
The simplest exponential inequalities with positive bases not equal to unity are solved in approximately the same way as similar equations. First, they need to try to bring to the same degree base, i.e. get inequality of the form:

After that you need to go to a rational inequality, given that this transition should be carried out as follows: if the base level is greater than one, then the inequality sign change is not necessary, and if the ground level is less than one, then you need to change the inequality sign on the opposite (that means change “less” to “more” or vice versa). At the same time, minus-plus signs do not need to be changed anywhere around the previously studied rules. We write mathematically what we get as a result of this transition:
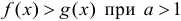

Next, you need to solve the standard rational inequality with all the subtleties of this procedure . The main thing to remember is that the inequality sign also changes when dividing the entire inequality by a negative number, or by an expression that takes negative values on the entire number axis. In this context, it is also useful to draw attention to the obvious fact: a positive number to any extent remains positive. And since we consider exponential inequalities with only positive grounds, all degrees in such inequalities are always positive.
More complex exponential inequalities can also be solved by changing variables. They can also be homogeneous; in this case, the replacement of the standard for homogeneous equations will be applied in exponential inequality, only with its help it will be necessary to solve the inequality.
If the exponential inequality cannot be reduced to a rational or solved by replacing, then in this case you need to apply the generalized interval method , which consists of the following:
- Determine DHS;
- Transform the inequality so that the right side has a zero (on the left side, if possible, lead to a common denominator, factor it out, etc.);
- Find all the roots of the numerator and denominator and put them on the number axis, moreover, if the inequality is weak, fill the roots of the numerator, well, leave the roots of the denominator in any case with punctured points;
- Find the sign of the whole expression on each of the intervals, substituting in the transformed inequality a number from this interval. In this case, it is no longer possible to alternately alternate signs passing through points on the axis. It is necessary to determine the sign of the expression on each interval by substitution of the value from the interval into this expression, and so for each interval. It can’t be done anymore (this is what, by and large, is the difference between the generalized method of intervals and the usual one);
- Find the intersection of the TLD and satisfying the inequality of the intervals, while not losing individual points that satisfy the inequality (the roots of the numerator in weak inequalities), and do not forget to exclude from the answer all the roots of the denominator in all inequalities.