What is an Irrational Equation? Definition, Rules with Examples
Basic theoretical information
In order to solve the tasks of this topic well, it is necessary to perfectly assimilate the theory from some previous topics, especially from the topics ” Irrational equations and systems ” and ” Rational inequalities “. We now write down one of the main theorems used in solving irrational inequalities (that is, inequalities with roots). So, if both functions f ( x ) and g (x) are non-negative, then the inequality:

Equivalent to the following inequality:

In other words, if the left and right inequalities are non-negative expressions, then this inequality can be safely raised to any degree. Well, if you want to raise all inequality to an odd degree, then in this case it is not necessary even to require the non-negativity of the left and right sides of the inequality. Thus, any inequality without restrictions can be raised to an odd degree. We emphasize once again that in order to raise the inequality to an even degree, it is necessary to make sure that both sides of this inequality are non-negative.
This theorem becomes very relevant precisely in irrational inequalities, i.e. in inequalities with roots, where, to solve most of the examples, it is necessary to raise inequalities to some degree. Of course, in irrational inequalities, it is necessary to carefully consider DHS, which is mainly formed from two standard conditions:
- the roots of even degrees must contain non-negative expressions;
- denominations of fractions should not be zeros.
Also, recall that the root value itself of an even degree is always non-negative.
In accordance with the above, if the irrational inequality has more than two square roots, then before squaring the inequality in the square (or another even power), you need to make sure that there are non-negative expressions on each side of the inequality, i.e. sums of square roots. If on one of the sides of the inequality there is a difference in the roots, then nothing can be known in advance about the sign of such a difference, and therefore it is impossible to raise the inequality to an even degree. In this case, you need the roots in front of which the minus signs are transferred to opposite sides of the inequality (from left to right or vice versa), thus the minus signs in front of the roots will change to “pluses” and only sums of roots will be obtained from both sides of the inequality. Only then can all inequalities be squared.
As in the other topics in mathematics, the method of changing variables can be used to solve irrational inequalities. The main thing to remember is that after introducing a replacement, the new expression should become simpler and not contain the old variable. In addition, you must not forget to perform a reverse replacement.
Let us dwell on several relatively simple but common types of irrational inequalities. The first type of such inequalities is when two roots of even degree are compared, i.e. there is an inequality of the form:
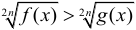
This inequality contains non-negative expressions on both sides, so it can be safely raised to the power of 2 n, after which, taking into account the LDL, we get
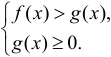
Notice that the TLD is written only for that smaller expression. Another expression will automatically be greater than zero since it is greater than the first expression, which in turn is greater than zero.
In the case when the root of an even degree is assumed to be greater than some rational expression, i.e. in the case when there is an irrational inequality of the form:
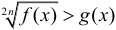
That solution to this inequality is accomplished by moving to a combination of two systems:

Finally, in the case when the root of an even degree is assumed to be less than some rational expression, i.e. in the case when there is an irrational inequality of the form:
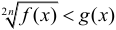
The solution to this inequality is made by moving to the system:

In cases where two roots of an odd degree are compared, or the root of an odd degree is assumed to be greater or less than some rational expression, you can simply raise all inequalities to the desired odd degree and thus get rid of all the roots. In this case, there is no additional DHS, since inequalities can be raised to an odd degree without restrictions, and expressions of any sign can stand under the roots of odd degrees.
Generalized Interval Method
In the case when there is a complex irrational equation that does not fall under any of the cases described above, and which cannot be solved by erection to a certain degree, you need to apply the generalized interval method, which consists of the following:
- Determine DHS;
- Transform the inequality so that the right side has a zero (on the left side, if possible, lead to a common denominator, factor it out, etc.);
- Find all the roots of the numerator and denominator and put them on the number axis, moreover, if the inequality is weak, fill the roots of the numerator, well, and leave the roots of the denominator in any case with punctured points;
- Find the sign of the whole expression on each of the intervals, substituting in the transformed inequality a number from this interval. In this case, it is no longer possible to alternately alternate signs passing through points on the axis. It is necessary to determine the sign of the expression on each interval by substitution the value from the interval into this expression, and so for each interval. It can’t be done anymore (this is what, by and large, is the difference between the generalized method of intervals and the usual one);
- Find the intersection of the TLD and satisfy the inequality of the intervals, while not losing individual points that satisfy the inequality (the roots of the numerator in weak inequalities), and do not forget to exclude from the answer all the roots of the denominator in all inequalities.
