Logarithmic equations examples and solutions

Solving Logarithmic Equations

1. Step 1: Use the rules of exponents to isolate a logarithmic expression (with the same base) on both sides of the equation.
2. Step 2: Set the arguments equal to each other.
3. Step 3: Solve the resulting equation.
4. Step 4: Check your answers. …
5. Solve.

Basic theoretical information

Logarithm properties

The logarithm definition is easiest to write mathematically:

The definition of the logarithm can be written in another way:

Note the limitations that apply on the base of the logarithm ( a ) and on the sub-logarithmic expression ( x ). In the future, these conditions will become important constraints for DHS, which will need to be taken into account when solving any equation with logarithms. So, now, in addition to standard conditions leading to restrictions on TLDs (positive expressions under the roots of even degrees, non-equality of the denominator to zero, etc.), the following conditions must also be taken into account:

• A logarithmic expression can only be positive.
• The base of the logarithm can only be positive and not equal to one.

Please note that neither the base of the logarithm nor the sub-logarithmic expression can be equal to zero. Also note that the logarithm value itself can take all possible values, i.e. logarithm can be positive, negative, and equal to zero. Logarithms have so many different properties that follow from the properties of degrees and the definition of the logarithm. We list them. So, the properties of logarithms:

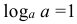
The logarithm of the product:

Logarithm fraction:

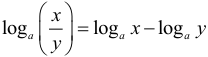
Extending the degree for the logarithm sign:

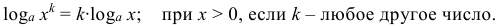
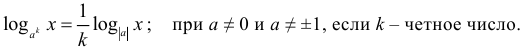
Pay particular attention to those of the last listed properties in which the module sign appears after the degree is rendered. Do not forget that when making an even degree for the logarithm sign, under the logarithm or at the base, you must leave the module sign.

Other useful logarithm properties:

The latter property is very often used in complex logarithmic equations and inequalities. He must be remembered as well as everyone else, although he is often forgotten about him.

Recommendations for solving logarithmic equations and systems

The simplest logarithmic equations are:

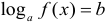
And their solution is given by the formula, which directly follows from the definition of the logarithm:

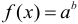
Other simplest logarithmic equations are those that can be reduced to the form using algebraic transformations and the above formulas and properties of logarithms:

The solution of such equations taking into account the TLD is as follows:

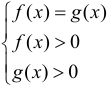
Some other logarithmic equations with a variable at the base can be reduced to the form:

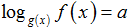
In such logarithmic equations, the general form of the solution also follows directly from the definition of the logarithm. Only in this case, there are additional restrictions for DHS that need to be taken into account. As a result, to solve a logarithmic equation with a variable at the base, the following system must be solved:

When solving more complex logarithmic equations that cannot be reduced to one of the above equations, the method of changing variables is also actively used. As usual, when applying this method, one should remember that after introducing a replacement, the equation should simplify and no longer contain the old unknown. Also, do not forget to perform the reverse substitution of variables.

Sometimes when solving logarithmic equations one also has to use the graphical method. This method consists in constructing graphs of functions on the same coordinate plane as functions on the left and right sides of the equation and then finding the coordinates of their intersection points in the drawing. Thus obtained roots must be verified by substitution into the original equation.

When solving logarithmic equations, the grouping method is often also useful. When using this method, the most important thing to remember is that: in order for a product of several factors to be equal to zero, it is necessary that at least one of them equals zero, and that the others exist. When the multipliers are logarithms or brackets with logarithms, and not just brackets with variables as in rational equations, many errors can occur. Since logarithms have many restrictions on the area where they exist.

When solving systems of logarithmic equations, it is often necessary to use either the substitution method or the variable replacement method. If there is such an opportunity, then when solving systems of logarithmic equations, one should strive to ensure that each of the equations of the system separately can be reduced to a form in which it will be possible to make the transition from a logarithmic equation to a rational one.

Recommendations for solving logarithmic inequalities

The simplest logarithmic inequalities are solved in approximately the same way as analogous equations. First, with the help of algebraic transformations and the properties of logarithms, they should be tried to bring to the form where the logarithms in the left and right sides of the inequality will have the same bases, i.e. get inequality of the form:

After that, you need to go to a rational inequality, taking into account that this transition should be done as follows: if the base of the logarithm is greater than one, then the inequality sign does not need to be changed, and if the base of the logarithm is less than one, then you need to change the inequality sign to the opposite (this means “less” to “more” or vice versa). At the same time, minus-plus signs do not need to be changed anywhere around the previously studied rules. We write mathematically what we get as a result of such a transition. If the base is greater than one we get:

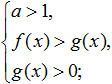
If the base of the logarithm is less than one, we change the inequality sign and we get the following system:

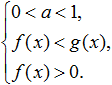
As you can see, when solving logarithmic inequalities, as well as the ADD is usually taken into account (this is the third condition in the systems above). Moreover, in this case, it is possible not to require the positivity of both sub-logarithmic expressions, and it suffices to require only the positive positivity of the smaller one.

When solving logarithmic inequalities with a variable at the base of the logarithm, it is necessary to independently consider both options (when the base is less than one and more than one) and combine the solutions of these cases as a whole. At the same time, it is necessary not to forget about DHS, i.e. about the fact that both the base and all sub-logarithmic expressions must be positive. Thus, in solving the inequality of the form:

We get the following set of systems:

More complex logarithmic inequalities can also be solved by changing variables. Some other logarithmic inequalities (as well as logarithmic equations) require the solution of the logarithmization of both parts of the inequality or equation on the same basis to solve. So when carrying out such a procedure with logarithmic inequalities there is subtlety. Note that with a logarithm over a base greater than one, the inequality sign does not change, and if the base is less than one, then the inequality sign changes to the opposite.

If the logarithmic inequality cannot be reduced to a rational or solved by replacing, then in this case you need to apply the generalized interval method, which consists of the following:

• Determine DHS;
• Transform the inequality so that the right side has a zero (on the left side, if possible, lead to a common denominator, factor it out, etc.);
• Find all the roots of the numerator and denominator and put them on the number axis, moreover, if the inequality is weak, fill the roots of the numerator, well, and leave the roots of the denominator in any case with punctured points;
• Find the sign of the whole expression on each of the intervals, substituting in the transformed inequality a number from this interval. In this case, it is no longer possible to alternately alternate signs passing through points on the axis. It is necessary to determine the sign of the expression on each interval by substitution the value from the interval into this expression, and so for each interval. It can’t be done anymore (this is what, by and large, is the difference between the generalized method of intervals and the usual one);
• Find the intersection of the TLD and satisfy the inequality of the intervals, while not losing individual points that satisfy the inequality (the roots of the numerator in weak inequalities), and do not forget to exclude from the answer all the roots of the denominator in all inequalities.