Plane Geometry Formulas

If we know the normal vector of a plane and a point passing through the plane, the equation of the plane is established. a ( x − x 1 ) + b ( y − y 1 ) + c ( z − z 1 ) = 0.

Geometry Formulas

Geometry formulas are used for finding dimensions, perimeter, area, surface area, volume, etc. of the geometric shapes. Geometry is a part of mathematics that deals with the relationships of points, lines, angles, surfaces, solids measurement, and properties. There are two types of geometry: 2D or plane geometry and 3D or solid geometry.

The 2D shapes are flat shapes that have only two dimensions, length, and width as in squares, circles, and triangles, etc. The 3D objects are solid objects, that have three dimensions, length, width, and height or depth, as in a cube, cuboid, sphere, cylinder, or cone, Let us learn geometry formulas along with a few solved examples in the upcoming sections.

Plane Geometry Formulas: Geometry can be divided into two different types: Plane Geometry and Solid Geometry. Plane Geometry deals with shapes such as circles, triangles, rectangles, squares,s, and more. Whereas, Solid Geometry is concerned with calculating the length, perimeter, area, and volume of various geometric figures and shapes.

Triangle

When solving problems in geometry, in addition to all the geometric formulas and properties that will be given below, it is necessary to remember very well the basic formulas for trigonometry. Let’s start with a few basic properties of different types of angles:

• Adjacent angles add up to 180 degrees.
• Vertical angles are equal to each other.

We now turn to the properties of the triangle. Suppose there is an arbitrary triangle:

Then, the sum of the corners of the triangle :

Remember also that the sum of any two sides of a triangle is always greater than a third party . The area of ​​the triangle through two sides and the angle between them:

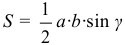
The area of ​​the triangle through the side and the height lowered onto it:

The triangle semi-perimeter is as follows:

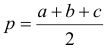
Heron’s formula for the area of ​​a triangle:

The area of ​​the triangle through the radius of the circumcircle:

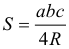
Median formula (median – a line drawn through a certain vertex and middle of the opposite side in a triangle):

Median properties:

• All three medians intersect at one point.
• The medians divide the triangle into six triangles of the same area.
• At the intersection point, the medians are divided by a ratio of 2: 1, counting from the vertices.

The bisector property (a bisector is a line that divides a certain angle into two equal angles, that is, in half):

It is important to know:  The center of a circle inscribed in a triangle lies at the intersection of bisectors (all three bisectors intersect at this one point). Formula bisector:

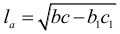
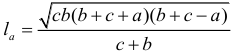
The main property of the heights of a triangle (height in a triangle is a line passing through a certain vertex of the triangle perpendicular to the opposite side):

All three heights in the triangle intersect at one point. The position of the intersection point is determined by the type of triangle:

• If the triangle is acute, then the point of intersection of the heights is inside the triangle.
• In a right triangle, heights intersect at the vertex of a right angle.
• If the triangle is obtuse, then the point of intersection of the heights is outside of the triangle.

Formula height:

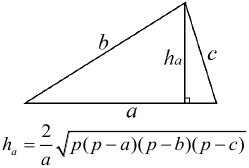
Another useful property of the heights of the triangle:

Cosine theorem :

Sinus Theorem :

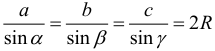
The center of the circle described near the triangle lies at the intersection of the middle perpendiculars. All three middle perpendiculars intersect at one this point. The middle perpendicular is the line drawn through the middle of the side of the triangle perpendicular to it.

The radius of a circle inscribed in a regular triangle:

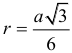
The radius of a circle described around a regular triangle:

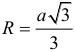
Area of ​​a right triangle:

Pythagorean theorem for a right triangle ( c – hypotenuse, a and b – legs):

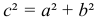
The radius of a circle inscribed in a right triangle:

The radius of a circle described around a right triangle:

The area of ​​a right triangle ( h is the height lowered to the hypotenuse):

Properties of the height lowered on the hypotenuse of a right triangle:

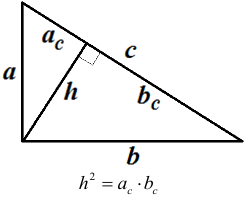
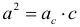
Such triangles are triangles whose angles are respectively equal, and the sides of one are proportional to the similar sides of the other. In such triangles, the corresponding lines (heights, medians, bisectors, etc.) are proportional. Similar sides of similar triangles are opposite sides of equal angles. The coefficient of similarity is the number k , equal to the ratio of the similar sides of similar triangles. The ratio of the perimeters of such triangles is equal to the coefficient of similarity. The ratio of the lengths of bisectors, medians, heights and median perpendiculars is equal to the coefficient of similarity. The ratio of the areas of similar triangles is equal to the square of the similarity coefficient. Signs of similarity of triangles:

• In two corners. If two corners of one triangle are respectively equal to two corners of another, then the triangles are similar.
• On two sides and the corner between them. If two sides of one triangle are proportional to two sides of another, and the angles between these sides are equal, then the triangles are similar.
• On three sides. If the three sides of one triangle are proportional to the three similar sides of the other, then the triangles are similar.

Trapezium

A trapezoid  is a quadrilateral in which exactly one pair of opposite sides is parallel. Trapezium centerline length:

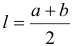
Trapezium area:

Some trapezoid properties:

• The middle line of the trapezoid is parallel to the bases.
• The segment connecting the centers of the diagonals of the trapezium is equal to the half-difference of the bases.
• In the trapezium of the midpoints, the intersection point of the diagonals and the intersection point of the side extensions are on the same line.
• The diagonals of a trapezoid divide it into four triangles. The triangles whose sides are bases are similar, and the triangles whose sides are sides are equal in size.
• If the sum of the angles at any base of the trapezium is 90 degrees, then the segment connecting the midpoints of the bases is equal to the half-difference of the bases.
• In an isosceles trapezoid, the angles for any base are equal.
• In an isosceles trapezoid, the diagonals are equal.
• In an isosceles trapezoid, the height lowered from the apex onto a larger base divides it into two segments, one of which is equal to the half-sum of bases, and the other half-difference of the bases.

Parallelogram

A parallelogram is a quadrilateral whose opposite sides are parallel in pairs, that is, they lie on parallel lines. The area of ​​the parallelogram through the side and the height lowered onto it:

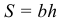
The area of ​​the parallelogram through two sides and the angle between them:

Some properties of the parallelogram:

• The opposite sides of the parallelogram are equal.
• The opposite angles of the parallelogram are equal.
• The diagonal parallelograms intersect and the intersection point is divided in half.
• The sum of the angles adjacent to one side is 180 degrees.
• The sum of all the corners of the parallelogram is 360 degrees.
• The sum of the squares of the parallelogram diagonals is equal to twice the sum of the squares of its sides.

Square

A square is a quadrilateral in which all sides are equal, and all angles are equal to 90 degrees. The area of ​​a square through the length of its side:

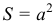
The area of ​​the square through the length of its diagonal:

The properties of a square are all properties of a parallelogram, a rhombus and a rectangle at the same time.

Rhombus and Rectangle

A rhombus is a parallelogram, in which all sides are equal. Diamond square (the first formula is two diagonals, the second is through the length of the side and the angle between the sides):

Diamond properties:

• The rhombus is a parallelogram. Its opposite sides are pairwise parallel.
• Diagonal rhombus intersect at right angles and at the intersection point are divided in half.
• Diagonal rhombus are the bisectors of its angles.

A rectangle is a parallelogram, in which all angles are right (equal to 90 degrees). The area of ​​the rectangle through two adjacent sides:

Rectangle properties:

• The diagonals of the rectangle are equal.
• A rectangle is a parallelogram – its opposite sides are parallel.
• The sides of the rectangle are at the same time its heights.
• The square of the diagonal of a rectangle is equal to the sum of the squares of its two not opposite sides (according to the Pythagorean theorem).
• A circle can be described near any rectangle, and the diagonal of the rectangle is equal to the diameter of the circumcircle.

Freehand shapes

The area of ​​an arbitrary convex quadrilateral in two diagonals and the angle between them:

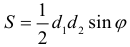
Connection of the area of ​​an arbitrary figure, its half-perimeter and the radius of the inscribed circle (it is obvious that the formula is valid only for figures in which a circle can be entered, that is, including for any triangles ):

The generalized theorem of Thales: Parallel straight lines cut off on proportional segments.

The condition under which it is possible to inscribe a circle in a quadrilateral:

The condition under which it is possible to describe a circle around a quadrilateral:

The sum of the angles of the n -gon:

Central angle of a regular  n -gon:

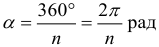
The area of ​​a regular  n -gon:

Circle

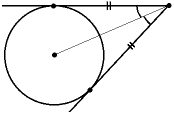
Tangent property:

Chord property:

The theorem on proportional segments of chords:

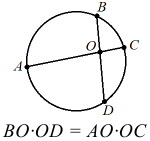
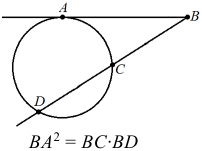
Theorem on tangent and secant:

The theorem of two secants:

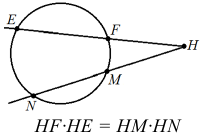
The theorem on the central and inscribed angles (the size of the central angle is twice the size of the inscribed angle, if they are based on a common arc):

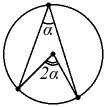
Property of inscribed angles (all inscribed angles based on a common arc are equal to each other):

Property of the central corners and chords:

Property of the central corners and secants:

Circumference :

Circumference:

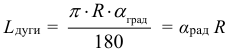
Circle Area :

Sector Area:

Ring area:

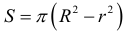
Circular segment area: